Physicist: Right around the end of the 19th century, Konstantin Tsiolkovsky (of rocket equation fame) got to thinking about how a taller version of the Eiffel tower, built on the equator, might be a good way to get things into orbit. It turns out that the craziest part of that idea isn’t the space part, it’s the tower part. If you want to build an elevator to space, you don’t put it in a tower, you hang it from the sky. Obviously.
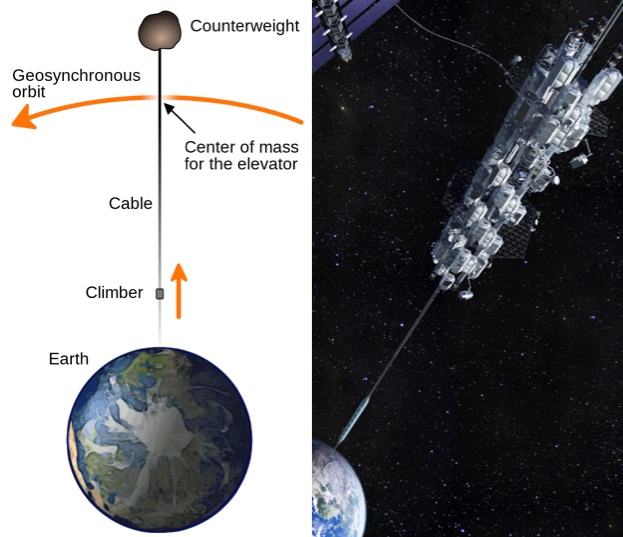
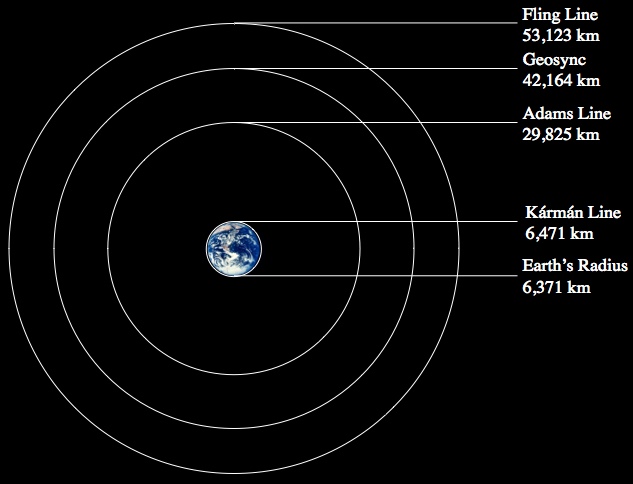
The basic set up for a space elevator. Above geosynchronous orbit, 42,000 km up, the tether will swing fast enough that it will “fall up”, keeping the whole system upright.
In order to build a space elevator we’d first send a bundle of cable to geosync (or manufacture it in space) and then lower one end to the ground. Once it’s anchored, we’d get new cables into place by running them up the old cables like a flag pole.
The cost of sending stuff to space has dropped precipitously in the last few years, as people of staggering genius discovered not-throwing-your-rocket-away technology. But even with reusable equipment, rocket flight has a number of drawbacks that are unlikely to ever be fixed. Rockets are powered by rocket fuel, which means that there’s a whole extra process for supplying them energy. And since a rocket needs to carry all the fuel it burns, the first (and largest) stage of a rocket is almost entirely fuel used to lift other fuel. And then of course are all of the usual issues that crop up when you ride an explosion into space. It’s good that the lower stages are now being reused, but it’s bad that they exist at all.

“The usual issues”
Vehicles that climb up and down a space elevator can be as small as you like, run on electricity, and don’t suffer from the burning/exploding snafus native to rocketry. While the trip will be much slower and controlled, more like travel by train than roller coaster, the real advantage of space elevators is infinite free energy forever and a safe, convenient way to get to and from the surface of Earth and literally anywhere else in the solar system. Since the tether is anchored to the ground, the rotation of the Earth supplies sideways velocity to anything attached to the tether and (like anything that rotates) that sideways velocity adds up to a lot if you get far enough out.
The higher something is, the longer it takes to orbit. Near the surface (within a few hundred km) orbits around the Earth take about 90 minutes, while the Moon (385,000 km away) takes about a month. One Lunar month in fact. In between is “geosynchronous orbit”, where objects can orbit the Earth in exactly one day, allowing them to stay over a single point on the equator indefinitely. If you ever see a satellite dish that’s nailed to a wall, it’s pointing at a satellite in geosync, because only those satellites sit perfectly still in the sky with respect to the ground. If you run a cable from the equator straight up, below geosync it will want to fall, because it will be traveling slower than orbital speed (that’s why ropes don’t stand up on their own), but above geosync it will want to fall up, because it will be traveling faster than orbital speed. If you swing a rope around over your head it will point straight away from you and for exactly the same reason space elevators stay upright (as long as enough of its mass is above geosync). Basically, space elevators turn Earth into a giant space weed wacker.
The big hurdles to building a space elevator are: 1) it sounds like a physicist stoner fantasy and 2) for Earth there are only a few things you can build a space elevator out of and we can’t manufacture any of them on the scales we need. The first problem is an old one: electricity, radios, computers, satellites, and practically every other important advancement were deemed impossible and pointless right up until they became easy and essential. The second problem is a bit more fundamental.
A hanging rope has to hold up its own weight in addition to whatever is tied to it. There’s a limit to how far a rope can hang before it snaps under its own weight, called the “breaking length”. Perfect name. The breaking length is a function of both the density of the material and the tensile strength; it’s greatest for materials that are light and strong. Unfortunately, the breaking lengths for our usual building materials is a little short. For example, the breaking length for steel is about 6.4 km.

These musical pipes haven’t snapped under their own weight because they’re shorter than 2.5 km, the breaking length of copper.
So why no just make the top of the cable thicker than the bottom? On the scale of sky scrapers that’s exactly what we do. But on the scale of the sky itself, it’s silly. Since the cable is pulled in both directions away from geosync, it needs to be thickest there; geosync is “the top of the hill”. By declaring that you’d like the tension to be constant along the entire cable (the best way to avoid snapping), you can derive how many times thicker it needs to be at geosync compared to its size at ground level (or wherever else you’d prefer). This is the “taper ratio”. Building a space elevator out of steel would entail a taper ratio of around 10175, meaning that a steel cable that’s 1 cm on the ground would have a diameter of around 1060 times the size of the observable universe at geosync, which would be difficult to construct for… any number of reasons. Carbon nanotubes on the other hand have a breaking length of around 6,000 km and a taper ratio of 1.6 between the ground and geosync. Even if you make the ratio 100, to accommodate extra equipment, lots of climbers, and a generous safety margin, that’s still totally feasible. It might look a little weird if you looked at the geosync station and the ground anchor at the same time, but you can’t: they’re 36,000 km apart.
The problem is that carbon nanotubes don’t come in 40,000+ km lengths. The record so far is about half a meter. That’s really impressive for a single molecule, but it would be nice to have intact strands stretching the full length of the tether, rather than tying or gluing them together (which introduces weak points). That right there is the big stumbling block for actually building space elevators: carbon nanotubes are presently too short and expensive. But that’s an engineering issue. We know we can do it, we just need to work out the details and then get good at it. In the last 20 years new fabrication techniques have massively increased the yield and dropped the price of nanotubes by a factor of more than 100. Recently, nanotube “yarn”, made from lots of short bits of nanotube, has demonstrated that it can handle about a third of the theoretical maximum, which is more than good enough for a space elevator (the taper ratio would be, gasp… 4.5ish).
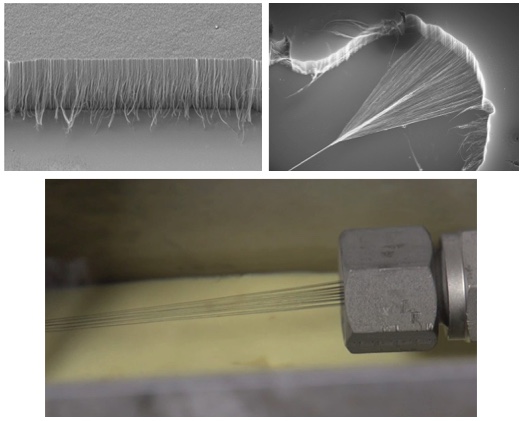
Left: Nanotubes typically aren’t manufactured, they’re “grown” through vapor deposition; you blow hot carbon over this forest, and the atoms that stick, usually stick in the right place. Right: But individual nanotubes generally don’t grow to be both flawless and long, so instead they can be gathered up as a kind of yarn with substantially worse performance. Bottom: That looks like this!
This report for NIAC (NASA Institute for Advanced Concepts and incomplete acronyms) goes through the problems, feasibility, and cost of a space elevator and comes up with a $40 billion price tag for the first elevator and a hell of a lot less for the second. Budget estimates like this are not the sort of thing that should be taken literally. “$40 billion” really means “somewhere between $4 and $400 billion”. The science of reading tea leaves and related sub-fields, like economics, are not great for exact predictions.
So what’s the point? In short, you personally will never go to the Moon or another planet by rocket. If you do go, it’ll be by elevator. Typically when you see a diagram of a space elevator, you see something like the image at the top of this article: Earth, geosynchronous orbit, and a space elevator extending just a little bit beyond it. But that tail above geosync is worth considering. Therein fun happens.

A space elevator doesn’t just sedately move material up and down. If you let go when you’re high enough, it also has the power to fling you across the solar system.
The higher you are the faster you’ll be moving and the less gravity you have to fight against. A space elevator to 45,000 km gives us access to Earth orbit. The savings from not needing rockets to put stuff in orbit means the elevator would pay for itself in short order, which is… fine. On the other hand, an elevator extending out to 200,000 km changes the course of human history. It gives us immediate and cheap access to and from everything in the solar system. Our world would go from dangerously small and crowded, to inconceivably vast and resourced in a single generation (unless there’s a reason to drag our feet). Just for some idea of where we might want to go, there’s likely to be more water on both Enceladus and Europa than on Earth and 16 Psyche is a brick of metallic iron the size of a state, which is especially appealing considering that Earth has zero natural metallic iron (it’s a long, polluting road from iron ore to iron). Easy access to effectively infinite resources along with plenty of room to grow is a plus.
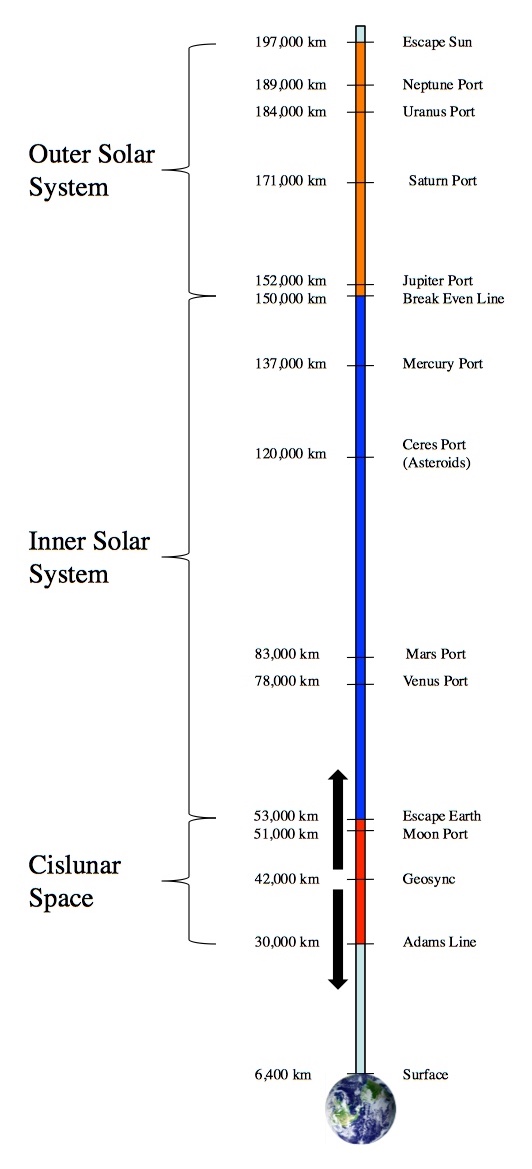
You can build ports to different locations around the solar system at different altitudes along the cable. Below 30,000 km you’d fall back to Earth. Between 30,000 and 53,000 km, you’d be in orbit around Earth. And above 53,000 km, you’d find yourself meandering about the solar system.
If you want to know how to throw a rock that would land on Mars in the least time, using the least energy, the answer is a “Hohmann transfer orbit“. When you hear about the launch window for Mars only being open once every 780 days (once per synodic period), that’s the window for the Earth-to-Mars Hohmann transfer orbit. Any other trajectory typically takes longer and requires a lot more rocket power at both ends.
A space elevator is like a sling for space craft and cargo. By climbing to the appropriate height and letting go during the appropriate launch window, you can go anywhere in the solar system without the need to lift off from Earth or work to escape its gravity. And the gains in efficiency that come from that are not small. That whole “rockets need to use fuel to carry their fuel” thing gets out of hand really fast. Freedom from lift-off means freedom from most of your rocket.
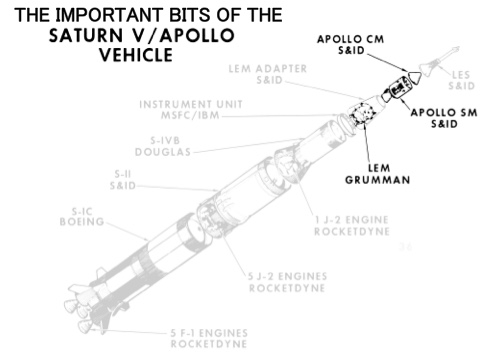
Practically all of every launch vehicle is wasted in the effort to escape Earth’s surface. This is a problem we can live without. The greyed-out stuff is everything we could scrap if we felt the need to repeat the Apollo Moon landings using a space elevator. All that’s left is the equipment for landing on and returning from the Moon (it’s a lot easier to go from there to here).
The Hohmann transfer orbits we use to get to each other planet, are the same ones we’d use to return. So the “Mars port” is also the best place to catch incoming stuff from Mars. Asteroid miners wouldn’t have to worry about safely landing cargo on Earth or even slowing it down; they’d just need to aim at the Ceres port and lower it from there. Earth is about the worst place to build a space elevator, which is why we need nanotubes to do it. You can easily do the same trick with rotating asteroids to fling stuff back to Earth using cables made from steel and suddenly you’ve got a free ride back home as well.
When designing space elevators, one of the big stumbling blocks often seems to be powering the climber to go up and down. The consensus seems to be that lasers are a good way to get power to a single climber going in a single direction (electrical cables conduct about as well as wood over tens of thousands of miles). But that’s very 20th-century-space-thinking. Consider this: counter weights.

Left: A metallic counter weight for an ordinary elevator. Right: 21 Lutetia, a source of metallic counter weights for a space elevator (enough to do that Apollo thing 57 trillion times). With enough counter weight, the energy needed to raise an elevator drops to zero. In other words, the more resources we ship to Earth, the lower the cost of leaving Earth.
If you’ve ever had the opportunity to look inside an open elevator shaft, you may have noticed that it’s more complicated in there than a single cable attached to the top of the elevator car. That’s because of the counter weight (and probably safety or something). The cable coming out of an elevator car goes over a pulley and attaches to a counter weight, so that as the elevator car rises the counter weight falls. For every 1 kg going down, you get 1 kg going up for free (or nearly free, what with friction). The same idea can be applied to space elevators. It takes a lot of power to get a ship up to the Mars port, but if there’s also a ship returning, then everyone gets a much cheaper ride. If there’s more cargo coming in from the asteroid belt than ships leaving Earth, then suddenly “counter weight tourism” begin to make sense. Rather than intentionally shedding energy to bring cargo to the ground, you may as well send stuff up. People, spaceships, garbage, Pog collections, whatever you got.
Almost as good, at 150,000 km, coincidentally between the ports for inner and outer solar system destinations, is the “break even line”. If you imagine the elevator as “uphill” from the ground to geosync and “downhill” after that, then the break even line is where you’ve gone uphill as much as you’ve gone down hill, so (ignoring friction and assuming the judicious use of counter weights) it takes a total of zero energy to get to the break even line and if you want to go to the outer solar system, there’s a net gain.
At just short of 200,000 km (just over halfway to the Moon) a space elevator can fling stuff into interstellar space, completely clear of the solar system. While that goes a long way toward traveling to other stars (we don’t have to worry about climbing out of the Sun’s gravity), on its own a space elevator won’t get us moving fast enough to go anywhere outside of the solar system on a even remotely reasonable time scale, if at all. For example, we recently had an interstellar interloper pass through the inner solar system, ‘Oumuamua. ‘Oumuamua is a rock traveling at a fairly typical clip of 26 km/s relative to the Sun when it’s in interstellar space. Unfortunately, to catch up with even this, the closest of all extra-solar objects, we’d need a tether that extends beyond the Moon (out to around 545,000 km). That presents two problems: first, the taper ratio jumps into the thousands even for nanotubes, and second, the Moon’ll smash the elevator. Still, “everywhere in the solar system for free” is better than a swift a kick in the pants.
To be clear, the space elevator described above is not crazy science fiction; it’s science fact that just doesn’t happen to exist right now. Even at the extreme end of the tether you’d experience a maximum of a tenth of Earth’s gravity, so you’re not exactly crushed by centrifugal forces, and the taper ratio never gets above about 2.5. This is something we can build and comfortably use. It would be a project on the scale of the Great Wall of China (21,196 km) or the Interstate Highway System (77,556 km), but it’s definitely doable.
Answer Gravy: It may have occurred to you that we live in 3 dimensional space. That means that places roughly in the plane of the equator are potential targets for a space-elevator-fling, but things above one of the poles are out of reach. The spin of the Earth keeps it oriented in space, like a gyroscope (well, not like a gyroscope) and that means that if you extend the equator out into space you’ll always hit the same line of stars across the sky, the “celestial equator”. Everything in the solar system orbits the Sun in roughly the same plane, and since the Earth is included in that plane it appears to us as another line across the sky, “the ecliptic”. With rare exceptions (mostly things orbiting way out there), everything in our solar system can always be found close to the ecliptic. These two planes are such important landmarks (spacemarks?) that their intersection is used to define 0° in celestial coordinates; kind of the “Greenwich of the sky”.
The ecliptic, the (green) path of the Sun and all the planets across the sky, is never more than about 23.5° away from the celestial equator, where an Earth-based space elevator can fling you directly.
So an important question before building a space elevator for the purpose of flinging is “How close are the celestial equator and the ecliptic?”. It turns out that they’re at most 23.5° apart. Not coincidentally, this is the Earth’s axial tilt (the ecliptic is what the Earth is tilted with respect to).
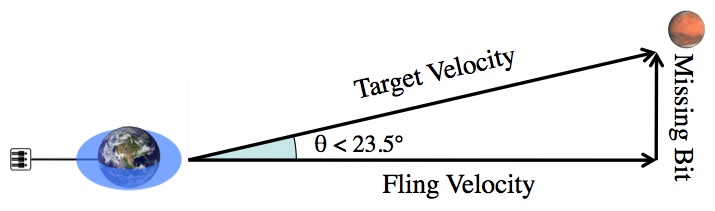
Mars (and basically everything else in the solar system) is on the ecliptic. Earth’s equator, and the arc of any space elevator, is tilted by about 23.5° with respect to the ecliptic.
If  ,
,  , and
, and 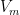 , are the target, fling, and missing velocities and if
, are the target, fling, and missing velocities and if  is the angle between the target velocity and the celestial equator, then by the Pythagorean theorem
is the angle between the target velocity and the celestial equator, then by the Pythagorean theorem  and by definition
and by definition  and
and  . The total energy the space craft needs is
. The total energy the space craft needs is  , and since
, and since  , the space elevator covers
, the space elevator covers  of the total energy cost. That means that in the worst case scenario about
of the total energy cost. That means that in the worst case scenario about  of the energy you need to get where you’re going is free. Considering that liftoff is no longer a going concern, this is a great opportunity for ion drives (much more efficient, but too weak for liftoff) to pick up the slack.
of the energy you need to get where you’re going is free. Considering that liftoff is no longer a going concern, this is a great opportunity for ion drives (much more efficient, but too weak for liftoff) to pick up the slack.
If you’re wondering how we can tell which materials are worth trying, it comes down to the usual physics process: say something reasonable, say it again with math, watch helplessly as the math spirals out of control, and finally (hopefully) learn something useful. If the stress on any part of the cable is especially high, then that’s where it’ll snap. To avoid weak points, we just need the entire cable to be under the same stress.
The total force that a cable can support is the cross-sectional area times the tensile strength (the maximum stress). This is because two cables can supply twice the force, so the total force must be proportional to the number of cables or (imagine bundling them together) the cross-section. Every elevation of the cable, r, supports the weight of the entire cable below it. As you rise up a tiny distance, dr, the cable now has to support the same weight, plus the additional weight of the cable between r and r+dr. So the additional area, dA, just needs to support the additional weight.
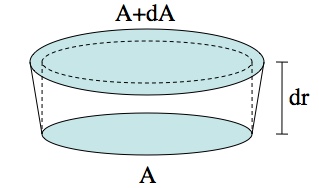
If you rise up a tiny distance, dr, the cable has to expand, dA, to handle the additional weight.
Weight is force times mass. Figuring out the mass is easy: it’s  , where
, where  is the density of whatever the cable is made of and
is the density of whatever the cable is made of and  is area times height, the volume of the “puck”. It may bother you that by ignoring the dA we’re pretending that the cable isn’t tapering. This is one of those big advantages of infinitesimals (and calculus in general). The volume we’re ignoring is
is area times height, the volume of the “puck”. It may bother you that by ignoring the dA we’re pretending that the cable isn’t tapering. This is one of those big advantages of infinitesimals (and calculus in general). The volume we’re ignoring is  . dA and dr are “infinitesimals”, meaning that (basically) they’re so small that things start to work out. As small as they each are, their product,
. dA and dr are “infinitesimals”, meaning that (basically) they’re so small that things start to work out. As small as they each are, their product,  , is a hell of a lot smaller. So ignore it. Things are working out.
, is a hell of a lot smaller. So ignore it. Things are working out.
“The additional area times the tensile strength is exactly enough to deal with the additional weight” written in mathspeak is
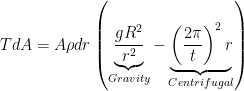
where T is the tensile strength,  is the acceleration of gravity on Earth’s surface,
is the acceleration of gravity on Earth’s surface, 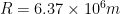 is the Earth’s radius, and
is the Earth’s radius, and  is the time it takes Earth to rotate once. We can simplify this a little by realizing that at the radius of geosync,
is the time it takes Earth to rotate once. We can simplify this a little by realizing that at the radius of geosync,  , these two forces are equal
, these two forces are equal
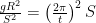
which means we can get rid of π (which is practically a variable, for all you can do with it) and t. Now: some algebra and calculus.
![\begin{array}{rcl} TdA &=& A\rho dr \left(\frac{gR^2}{r^2} - \frac{gR^2r}{S^3}\right) \\[2mm] TdA &=& A\rho gR^2dr \left(\frac{1}{r^2} - \frac{r}{S^3}\right) \\[2mm] \frac{1}{A}dA &=& \frac{\rho gR^2}{T} \left(\frac{1}{r^2} - \frac{r}{S^3}\right)dr \\[2mm] \int\frac{1}{A}dA &=& \int\frac{\rho gR^2}{T} \left(\frac{1}{r^2} - \frac{r}{S^3}\right)dr \\[2mm] \int\frac{1}{A}dA &=& \frac{\rho gR^2}{T}\int \left(\frac{1}{r^2} - \frac{r}{S^3}\right)dr \\[2mm] \ln(A)+C &=& \frac{\rho gR^2}{T}\left(-\frac{1}{r} - \frac{r^2}{2S^3}\right)+C \\[2mm] \ln(A) &=& -\frac{\rho gR^2}{T}\left(\frac{1}{r} + \frac{r^2}{2S^3}\right)+C \\[2mm] A &=& e^{-\frac{\rho gR^2}{T}\left(\frac{1}{r} + \frac{r^2}{2S^3}\right)+C} \\[2mm] A &=& Ce^{-\frac{\rho gR^2}{T}\left(\frac{1}{r} + \frac{r^2}{2S^3}\right)} \\[2mm] \end{array} \begin{array}{rcl} TdA &=& A\rho dr \left(\frac{gR^2}{r^2} - \frac{gR^2r}{S^3}\right) \\[2mm] TdA &=& A\rho gR^2dr \left(\frac{1}{r^2} - \frac{r}{S^3}\right) \\[2mm] \frac{1}{A}dA &=& \frac{\rho gR^2}{T} \left(\frac{1}{r^2} - \frac{r}{S^3}\right)dr \\[2mm] \int\frac{1}{A}dA &=& \int\frac{\rho gR^2}{T} \left(\frac{1}{r^2} - \frac{r}{S^3}\right)dr \\[2mm] \int\frac{1}{A}dA &=& \frac{\rho gR^2}{T}\int \left(\frac{1}{r^2} - \frac{r}{S^3}\right)dr \\[2mm] \ln(A)+C &=& \frac{\rho gR^2}{T}\left(-\frac{1}{r} - \frac{r^2}{2S^3}\right)+C \\[2mm] \ln(A) &=& -\frac{\rho gR^2}{T}\left(\frac{1}{r} + \frac{r^2}{2S^3}\right)+C \\[2mm] A &=& e^{-\frac{\rho gR^2}{T}\left(\frac{1}{r} + \frac{r^2}{2S^3}\right)+C} \\[2mm] A &=& Ce^{-\frac{\rho gR^2}{T}\left(\frac{1}{r} + \frac{r^2}{2S^3}\right)} \\[2mm] \end{array}](//s0.wp.com/latex.php?latex=%5Cbegin%7Barray%7D%7Brcl%7D+TdA+%26%3D%26+A%5Crho+dr+%5Cleft%28%5Cfrac%7BgR%5E2%7D%7Br%5E2%7D+-+%5Cfrac%7BgR%5E2r%7D%7BS%5E3%7D%5Cright%29+%5C%5C%5B2mm%5D+TdA+%26%3D%26+A%5Crho+gR%5E2dr+%5Cleft%28%5Cfrac%7B1%7D%7Br%5E2%7D+-+%5Cfrac%7Br%7D%7BS%5E3%7D%5Cright%29+%5C%5C%5B2mm%5D+%5Cfrac%7B1%7D%7BA%7DdA+%26%3D%26+%5Cfrac%7B%5Crho+gR%5E2%7D%7BT%7D+%5Cleft%28%5Cfrac%7B1%7D%7Br%5E2%7D+-+%5Cfrac%7Br%7D%7BS%5E3%7D%5Cright%29dr+%5C%5C%5B2mm%5D+%5Cint%5Cfrac%7B1%7D%7BA%7DdA+%26%3D%26+%5Cint%5Cfrac%7B%5Crho+gR%5E2%7D%7BT%7D+%5Cleft%28%5Cfrac%7B1%7D%7Br%5E2%7D+-+%5Cfrac%7Br%7D%7BS%5E3%7D%5Cright%29dr+%5C%5C%5B2mm%5D+%5Cint%5Cfrac%7B1%7D%7BA%7DdA+%26%3D%26+%5Cfrac%7B%5Crho+gR%5E2%7D%7BT%7D%5Cint+%5Cleft%28%5Cfrac%7B1%7D%7Br%5E2%7D+-+%5Cfrac%7Br%7D%7BS%5E3%7D%5Cright%29dr+%5C%5C%5B2mm%5D+%5Cln%28A%29%2BC+%26%3D%26+%5Cfrac%7B%5Crho+gR%5E2%7D%7BT%7D%5Cleft%28-%5Cfrac%7B1%7D%7Br%7D+-+%5Cfrac%7Br%5E2%7D%7B2S%5E3%7D%5Cright%29%2BC+%5C%5C%5B2mm%5D+%5Cln%28A%29+%26%3D%26+-%5Cfrac%7B%5Crho+gR%5E2%7D%7BT%7D%5Cleft%28%5Cfrac%7B1%7D%7Br%7D+%2B+%5Cfrac%7Br%5E2%7D%7B2S%5E3%7D%5Cright%29%2BC+%5C%5C%5B2mm%5D+A+%26%3D%26+e%5E%7B-%5Cfrac%7B%5Crho+gR%5E2%7D%7BT%7D%5Cleft%28%5Cfrac%7B1%7D%7Br%7D+%2B+%5Cfrac%7Br%5E2%7D%7B2S%5E3%7D%5Cright%29%2BC%7D+%5C%5C%5B2mm%5D+A+%26%3D%26+Ce%5E%7B-%5Cfrac%7B%5Crho+gR%5E2%7D%7BT%7D%5Cleft%28%5Cfrac%7B1%7D%7Br%7D+%2B+%5Cfrac%7Br%5E2%7D%7B2S%5E3%7D%5Cright%29%7D+%5C%5C%5B2mm%5D+%5Cend%7Barray%7D&bg=ffffff&fg=000&s=0)
Those C’s are constants of integration and, being unspecified numbers, you can do some pretty sloppy math with them: C ± C = C, eC = C, that sort of thing. If you set that last C to  , then you’ve declared that the cable has a maximum cross-section at geosync of 1 (units TBD). You can find values for the density and tensile strength of different materials here and you can graph them here, if you want to consider their feasibility for yourself.
, then you’ve declared that the cable has a maximum cross-section at geosync of 1 (units TBD). You can find values for the density and tensile strength of different materials here and you can graph them here, if you want to consider their feasibility for yourself.
. What this is saying (in mathspeak) is that after every half-life, the probability that an atom will remain is halved. For example, at t=2h (after two half-lives),
.
.
.