Physicist: To demonstrate this you typically have to use either calculus or oranges. They both use more or less the same ideas, they’re just applied in different ways.
Oranges:
Imagine taking an orange wedge and opening it so that the triangles all point “up” instead of towards the same point. If you interlaced two of these then you’d have a small brick that’s roughly rectangular.
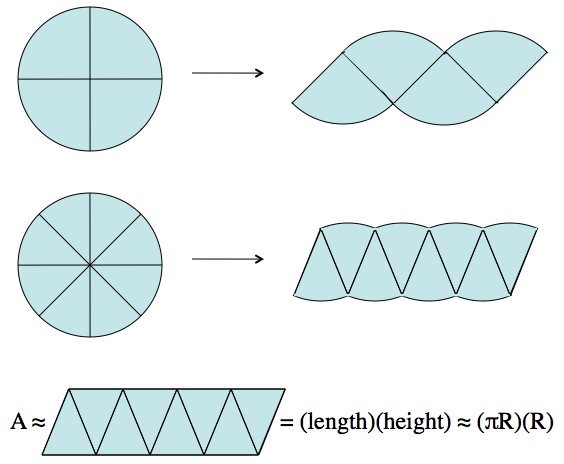
By slicing the circle with thinner and thinner triangles, the lines on the “wavey shape” all straighten out into a rectangle.
As more triangles are used, the curved end produces less pronounced bumpiness and the straight sides come closer and closer to being straight up and down, making the brick rectangular. The height becomes equal to the radius, while the length is half of the circumference (C = 2πR) which now finds itself running along the top and bottom. As the number of triangles “approaches infinity” the circle can be taken apart and rearranged to fit almost perfectly into an “R by πR” box with an area of πR2.
This is why calculus is so damn useful. We often think of infinity as being mysterious or difficult to work with, but here the infinite slicing just makes the conclusion infinitely clean and exact: A = πR2.
Calculus:
On the mathier side of things, the circumference is the differential of the area. That is; if you increase the radius by “dr”, which is a tiny, tiny bit, then the area increases by Cdr where C is the circumference. We can use that fact to describe a disk as the sum of a lot of very tiny rings. “The sum of a lot of tiny _____” makes mathematicians reflexively say “use an integral“.
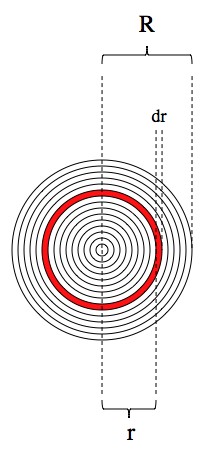
With calculus, you can find the area of a circle by adding up the areas of a lot (infinite number) of very thin (infinitely thin) rings. Here a random intermediate ring is red.
Every ring has an area of Cdr = (2πr)dr. Adding them up from the center, r=0, to the outer edge, r=R, is written: .
This is a beautiful example of understanding trumping memory. A mathematician will forget the equation for the area of a circle (A=πR2), but remember that the circumference is its differential. That’s not to excuse their forgetfulness, just explain it.







hello 🙂
http://oi60.tinypic.com/24nmgw0.jpg
Beautiful orange example.
I’m currently teaching set 1 year 3 this
A = πr2
A = 3.14 x radius x radius
A = answer
—- (If the radius isn’t shown then apply this rule before the area rule) —-
r = D/2
r = diam/2
r = answer
If Pi is an infinite number and the circumference of a circle is C = Pi r squared then how can a circle ever be complete?
@Dennis Hirsch:
By definition, C/d=pi. Thus the circle cannot be incomplete.
NICE PIC.
why only pi is taken when there would be infinite triangles
Shivendra Singh,
There would be an infinite amount of triangles. The curved side of the triangles will all add up to the circumference, which is equal to 2pi*r, however, since the sides merged together yield a circle. Because half of the triangles compose the width of the hypothetical rectangle, this makes the width equal to pi*r. That means, only pi is taken because the circumference is equal to 2*pi*r, which is where pi comes from.
Now that is what i call ‘giving perspective’.Loved your example which completely points to the fact that our nature and math rules are coherent.
Wow I see it and understand the individual words but as a whole it escapes me
Seems to me the triangles are not really triangle but “infinitely nearing” triangles. So A=LH is not quite the right formula. Should be something like “A=LH minus infinity small.” It is incomplete.
On the other hand, (Pi r)(r) completes the circle because pi is involved.
Said another way, saying A=LH = (Pi r)(r) is only true in the sense that 0.9999999… =1.
We just say those are the same thing but that has yet to be seen.
Troy,
A = LH becomes the correct formula after the difference is taken to arbitrarily near zero via limits, which is what needs to be done anyway since there is an infinite number of triangles. A = LH is correct, but understanding that requires calculus. So I personally think that the orange method is in itself just an application of calculus. I prefer the method of using coordinate planes rather than relying on the circumference formula, which is also derived from integration anyway.
When Math become simple thing
ahhh….the way you explained it was just beautiful……tysmm
what is the answer
Why is πr the length of the rectangle.
Ridgewood military school pictures
Should not be lost or stolen A(BC)=(AB)C
770-1974
(805-357-1165)
105-55