Physicist: This isn’t a question anybody asked, just an interesting goings-on.
A few weeks ago QUESS (QUantum Experiments at Space Scale) began teleporting quantum information to and from the Micius satellite and between ground stations 1200 km apart. This is exciting, because it demonstrates the feasibility of easy (cheap), high-fidelity, long-distance quantum entanglement, which is the key to all quantum communication. Micius is the first shaky pillar of a global-scale quantum infrastructure.
Time-lapse of a laser in Xinglong talking to Micius, the world’s first “quantum satellite”.
Entanglement is basically a combination of correlation and superposition. The difference between a bit and a qubit is that a bit is either 1 or 0 while a qubit is simultaneously 1 and 0. There are a lot of different forms that a qubit can take (just like there are many forms a bit can take): in this case the polarization of light is used. There are two possible polarization states, which is perfect for encoding two possibilities, 0 and 1 (and incidentally perfect for making 3D movies; one movie for each polarization and each eye).
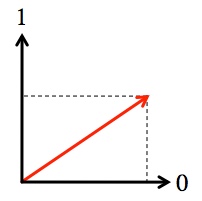
The polarization of light can point in any direction (perpendicular to the direction of travel), so we can use it to describe not just 0 or 1 but a combination of both.
A photon can be in a superposition of both horizontal and vertical polarization. When measured they are always found to be in one state or the other (0 or 1), but there are a lot of clever things we can do with qubits before doing that measurement.
While it is impossible to be sure of the result, the probability that you see a “0” or “1” is described by the state (in the picture above, “0” is more likely, but not guaranteed). The spooky thing about entangled particles is that, as long as you measure them the same way, their random results will be correlated. For the simplest kind of entangled state, 
How do you get entangled particles thousands of km apart? Carefully.
What QUESS is doing, and what “quantum communication” is all about, is getting two entangled particles far apart without accidentally breaking the entanglement or losing the particles (which is difficult when they’re being fired at you from space).
Once two widely separated parties share a pair of entangled particles, they can start doing some rather remarkable things. One of those is the ability to send qubits from one particle to its entangled twin: “quantum teleportation”. Quantum teleportation requires both an entangled pair of particles and a “classical communication channel” (which includes, but is not limited to, shouting loudly). With those in hand, we can easily “teleport” a qubit, from one location to another.
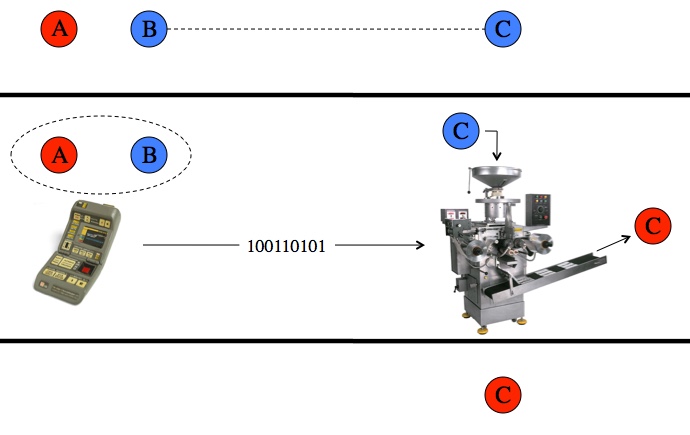
Top: One state you’d like to teleport, A, and two particles sharing an entangled state, B and C. Middle: Some relative properties of A and B are measured and the results are sent to whomever has the other entangled particle. Based on that information, the other entangled particle is manipulated. Bottom: The result is that the entanglement is destroyed, but C assumes A’s original state.
Qubits (quantum states in general) are as delicate as delicate can be. Absolutely any interaction capable of allowing anything to determine their state “collapses” that state; a qubit goes from being both 0 and 1 to being either 0 or 1, and all of the advantages that may have gone with that superposition go out the window. So teleportation needs to be able to measure the to-be-sent qubit, A, without actually determining anything about it, which is tricky. The way to get around that is to do a measurement that compares A and B, without measuring either of them directly. If you learn that two coins have either the same or opposite side up, then you’ve learned something about the two of them together, but nothing specific about either of them individually.
The same idea applies in quantum teleportation. The central idea behind entanglement is that if B and C are entangled, then they react to measurements in the same way. So by comparing A and B and learning how they’re different, you’re also learning how A and C are different. Knowing that, you can figure out what needs to be done to C to make it have the same state as A. And all without actually learning what that state is. Even if C is, hypothetically, on the far side of China, you can just tell whoever has it what the results of the test were. For coins/regular bits you only need to send one bit of information; the result of the comparison is either “same” or “different”. For qubits you need to send two bits, because of how terrifyingly complex quantum mechanics is. Here’s a bit more detail on how quantum teleportation works.
Not a lot of physicists are too surprised that ground-to-space quantum teleportation works (nobody builds and launches spacecraft on a hunch). There’s never been any indication that distance is a factor for quantum entanglement, so this isn’t a matter of overcoming physical laws, just getting around (a lot of) engineering difficulties. Teleportation is easy to do with equipment on opposite sides of a room. The difference here is that the “opposite side of the room” is moving at about 8 km/s and is in freaking space.
Quantum states are delicate, so we need to be able to catch, manipulate, and accurately measure the states of individual photons with minimal interference. Assuming that you’re not having someone else read this out loud, you are presently noticing that photons carry information through air pretty well. Pretty well. Over a large enough distance, even clean air is effectively opaque. The present through-air record for this same procedure is 143 km, between a couple of Canary Islands. That’s 143 km through the densest region of our atmosphere (sea level). There’s about as much air between you and space as there is between you and anything 7 km away along the ground (it gets thin fast as you go up). So teleportation straight up should be easier than teleportation between ground stations.
Generally speaking, the big problem with conveying intact quantum information is all the stuff in the way, so space is kind of an obvious solution. The problem with space is the distances involved; the farther something is away, the smaller the target. Establishing entanglement between two locations comes down to creating a pair of entangled particles in one location and then sending one of the pair to the other location. QUESS manages to catch about 1 in every 6 million photon pairs and it doesn’t work during the day because the sunlight scatters off of the air (likely to be a non-issue between two quantum satellites). In all, the QUESS team claims to be able to establish 1 entangled pair per second. All things considered, that’s bragging-rights-impressive. This and this is what constitutes “bragging” (the QUESS team’s official papers on the subject).
Even with a noisy channel, with lots of photons lost and the states of many of the others perturbed by their journey, a reliable quantum channel is still possible. We can distill quantum entanglement, turning many weakly entangled pairs into fewer strongly entangled pairs. You can think of this like repeating a digital message to get it across a noisy channel; it takes more time to send the signal, but the result is a message clearer than any of the individual attempts. Once an entanglement has been established between two parties, a quantum state can be teleported between the two, including a state entangled with something else. In this way, two entangled pairs between A-B and B-C can be turned into one entangled pair between A-C. With “quantum repeaters” in place, we can establish quantum channels over huge distances by piecing together many short, possibly noisy, channels. Point is: despite quantum states being perfectly delicate, we don’t need perfect delicacy to work with them.
In the golden age of the telegraph, we could send information (bits) anywhere, we just couldn’t do too much with them when they got there. We’re entering a similar (but likely to be much shorter) age of quantum information.
Quantum information technology is still in its infancy. Where we are now is analogous to the age of telegraphs and Morse code. We can send qubits, a few at a time, over long distances, but we don’t have computers at the ends capable of doing much with those qubits. Despite that massive shortcoming, there are some killer apps that are likely to drive this technology forward. In particular: quantum cryptography.
Skirting the details, quantum encryption boils down to:
1) distribute lots of pairs of entangled particles
2) measure each pair the same way
3) write down the results
No quantum computation involved! The defining characteristic of maximally entangled pairs is that measurements on the pair are perfectly correlated and fundamentally random. Anyone/thing that intercepts an entangled particle breaks (or at least weakens) the entanglement, so eavesdropping can be detected. For you cypherpunks out there, quantum cryptography is a method of creating a shared random secret that is perfectly robust to man-in-the-middle attacks (or at least detects such attempts). You and someone else create a random number that only the two of you can possibly know, which allows you to then encrypt any message and send it (by email for example) with security guaranteed by physical laws.
Quantum cryptography: sharing and securing secrets with fundamental physics!
Shockingly, this is of interest to lots of space-capable governments, so Micius is unlikely to be the last quantum communication satellite.
Just as a quick aside, since it’s often not stated clearly: quantum teleportation does not involve actual teleportation in any sense. Nothing actually makes the journey from one quantum system to the other. There are a pair of theorems in the field of quantum information theory that say that if you and someone else share an entangled pair of particles (sometimes called an “ebit”), then at the cost of that entanglement and the application of some cute tricks you can:
1) send 2 bits to convey one qubit
2) send 1 qubit to convey two bits
The first procedure is called “teleportation” and the second is called “super-dense coding”. One of those is a terrible, misleading name and the other is “super-dense coding”.
The laser picture is from here, the telegraph picture is from here, and the spy picture is by Tomer Hanuka.
>One of those is a terrible, misleading name
Dear Physicist,
Hooray for you and clarity!Teleportation is misleading hype.
Once upon a time at SLAC the publicity people put out a press release about our discovery at BaBar experiment about our discovery of CP violation in B-meson decays. It gave the impression we had explained why matter dominates over matter in the universe. Besides discovering CP violation and confirming the standard model, we actually discovered that CP violation in B-decay does NOT explain the matter-anti-matter asymmetry.
If you read the press release carefully, it was not actually wrong about this, but was worded to mislead and hype up the result.
Only the Economist got it right
From, “Once two widely separated particles” on through the illustrations, I was lost. Could you re say that in English as simply as you can. Thanks
@Tom Hendricks
I just read over it again and, yeah, the learning curve was a little too steep. I’ve put in a few more paragraphs to introduce entanglement and would love to know if I’m any closer to comprehensibility.
How long did it take to transmit the info, was it instantaneous as projected or take some time or couldn’t be measured. Thanks.
@George
However much time it takes to measure some particles here, send the results to the distant particle’s location, and to perform some manipulations on that distant particle. So quantum teleportation is slightly slower than email.
George: the information still can not get there faster then light. What entanglement gives you is a means to detect if somebody is peeking — nothing more. Indeed presumably a bit less — at least has I understand, it part of the bandwidth has to be used to measure Bell inequalities that allow you to check for spies.
My understanding was that when the state of the observed photon was observed, the other would be the same instantaneously. I was asking if the state changed instantaneously as I understood the photons could be light years a part and this would still be true. I though this instantaneous information transmitting was part of entanglement.
thanks
@George
This is part of how entanglement is like correlation. Say you and a friend have identical looking wallets and, after splitting a bill somewhere, you go your separate ways. When you get home you’ll know, by looking at the wallet in your hand, whether or not you friend has your wallet or their own. Instantly. But that doesn’t mean that they’re somehow connected.
With quantum entanglement there is no way whatsoever to tell if the other entangled particle has been measured or what that result was. All you can say is “when both have been measured, we will find that the results are the same”.
Thank you. I need to read up on this as I clearly misunderstood the part about using this as means of transmitting a message instantaneous over a distance. I appreciate the time and the insight.
@George
It’s not just you. “Instantaneous signal” shows up pretty regularly in the news.
If you send an entangled pair to different detectors, the correlation goes along for the ride. It is there all the time the photons are traveling. When the results of the measurement from one detector, arrive at the other you’ll be able to decode the message encoded in the entanglement instantly, but those results, needed to decode the message, will have been sent at the speed of light and the entangled photons carry the correlation never exceed the speed of light either.
You can know the result of measurement at one end by observing that at the other, but only by a prior agreement not to change the measurement settings on the detectors. If the guy at the other end changes his settings, you don’t know what result he’ll be getting.
Quantum Mechanics is very difficult to understand and highly counter-intuitive, as well as being central to a number of major philosophical and technological questions. This is pretty much the worst possible combination for dissemination to the general public, as it greatly encourages the propagation of misinformation.
People want to understand Quantum Mechanics, but they don’t want to spend years of study and research to do so. Unfortunately the nature of the field of study means you can’t really do that.
Still having trouble – I’ve narrowed it down to the 2nd illustration caption,
“Middle: Some relative properties of one entangled particle and the to-be-teleported particle are measured and the results are sent to whomever has the distant particle. Based on that information, the other entangled particle is manipulated. ”
Perhaps you could give specifics, Electron A has what information, how is it sent, to whom, where?
@Tom Hendricks
I went back and forth about whether the details of that would be more enlightening or time consuming and intimidating.
There are several different teleportation schemes depending on the nature of the quantum information to be sent. For a single qubit, the to-be-sent state and one of the entangled particles are measured in the “Bell basis” and since there are four Bell states (four possible results) the information sent is a number: 1, 2, 3, or 4.
The link right above that illustration leads to an old post that goes into detail. I’ll see if there’s a good way to fix this.
@Traruh Synred:
“>One of those is a terrible, misleading name
Dear Physicist,
Hooray for you and clarity!Teleportation is misleading hype.
Once upon a time at SLAC the publicity people put out a press release about our discovery at BaBar experiment about our discovery of CP violation in B-meson decays. It gave the impression we had explained why matter dominates over matter in the universe. Besides discovering CP violation and confirming the standard model, we actually discovered that CP violation in B-decay does NOT explain the matter-anti-matter asymmetry.
If you read the press release carefully, it was not actually wrong about this, but was worded to mislead and hype up the result.
Only the Economist got it right.”
This is incorrect. In fact, teleportation is the correct name because 1) there is no medium by which the information gets from A to B & 2) the information does not use the space-time fabric to travel. Information CAN be teleported using quantum entanglement, because as soon as the properties of the particle are measured, the properties of the entangled correspondent are immediately determined. The information itself will not be received faster than what light can travel through the distance between particles because measurements & signalling take significantly longer, but the information of the properties itself is determined regardless of distance and period.
“If you send an entangled pair to different detectors, the correlation goes along for the ride. It is there all the time the photons are traveling. When the results of the measurement from one detector, arrive at the other you’ll be able to decode the message encoded in the entanglement instantly, but those results, needed to decode the message, will have been sent at the speed of light and the entangled photons carry the correlation never exceed the speed of light either.
You can know the result of measurement at one end by observing that at the other, but only by a prior agreement not to change the measurement settings on the detectors. If the guy at the other end changes his settings, you don’t know what result he’ll be getting.”
Exactly. Hence the name “teleportation.” Teleportation need not refer to particles or energy.
If you define teleportation that way fine. A rather technical definition. It’s not what the public thinks the word means and thus misleading hype. There word is borrowed from SciFi where indeed it means teleportation stuff. “Beam me up Scotty”
Where is the information transfer gain in quantum teleportation if you still need a classical message? Is it like transferring a compressed file via Internet – the gain is in compression, not the speed?
@Kamil
There is a technique called “super dense coding”, which is the other side of the coin to “quantum teleportation”. In both techniques the two parties start with an entangled qubit. For quantum teleportation, two classical bits are sent and that’s enough to use the entangled pair to convey a qubit. For super dense coding, a qubit is sent and along with the entangled bit, that’s enough to convey two bits.
But the point isn’t speed or compression: it’s to send qubits, which are fundamentally different from bits. A bit is a one specific value out of two (typically called “0” or “1”, but “on” or “off” or any other binary choice works as well). A qubit is a specific combination of both values at the same time. Right now the only real use for sending qubits is “quantum key distribution” (quantum cryptography). But if/when quantum computers become scalable and useful, we’ll need to be able to send qubits for them to work together.